Manduca Sexta

Muscle Performance in a Soft-Bodied Terrestrial Crawler

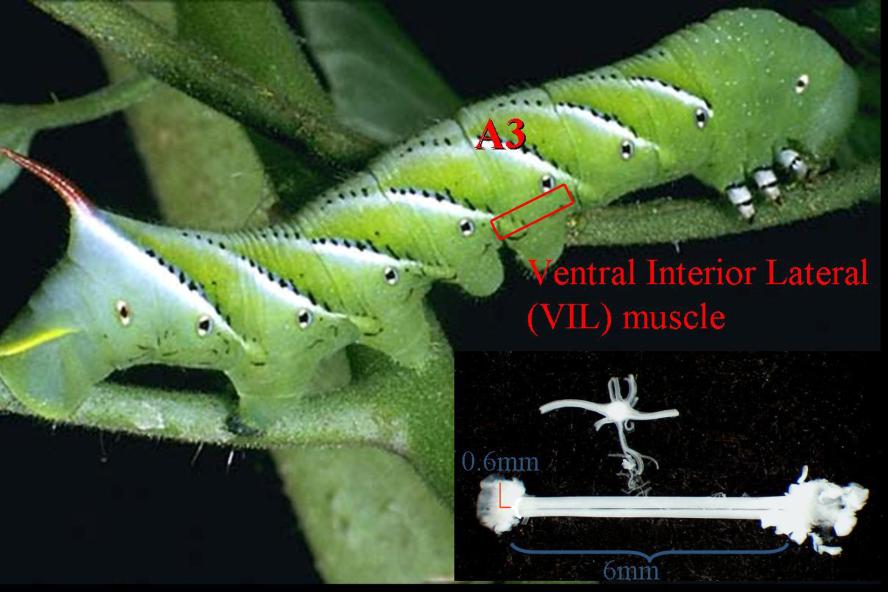
Figure 1: A fifth instar Manduca sexta caterpillar illustrating the position of the ventral internal lateral muscle in the third abdominal body segment.

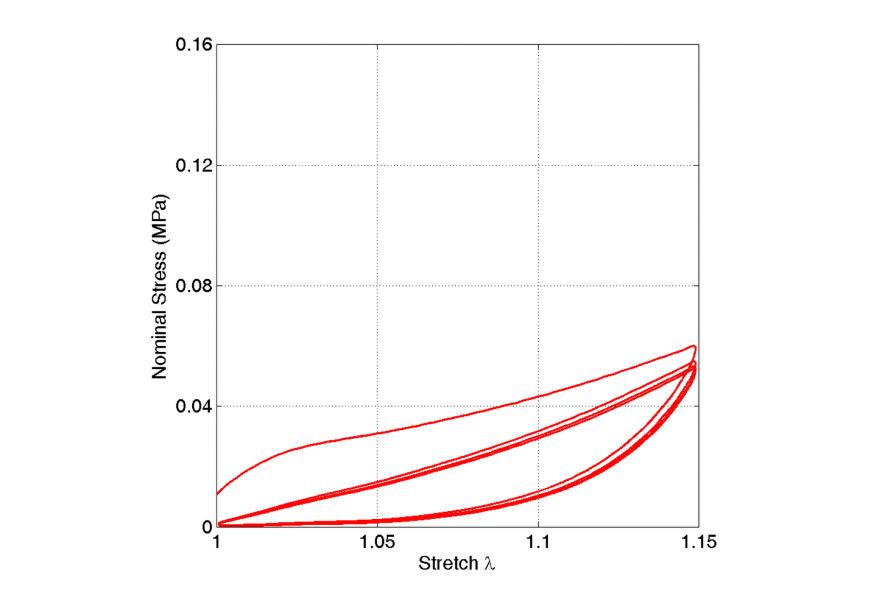
chart showing the pre-conditioning of a caterpillar muscle in the passive state with maximum stretch of 1.15

Figure 2: Pre-conditioning of a caterpillar muscle in the passive state with maximum stretch of λ = 1.15.

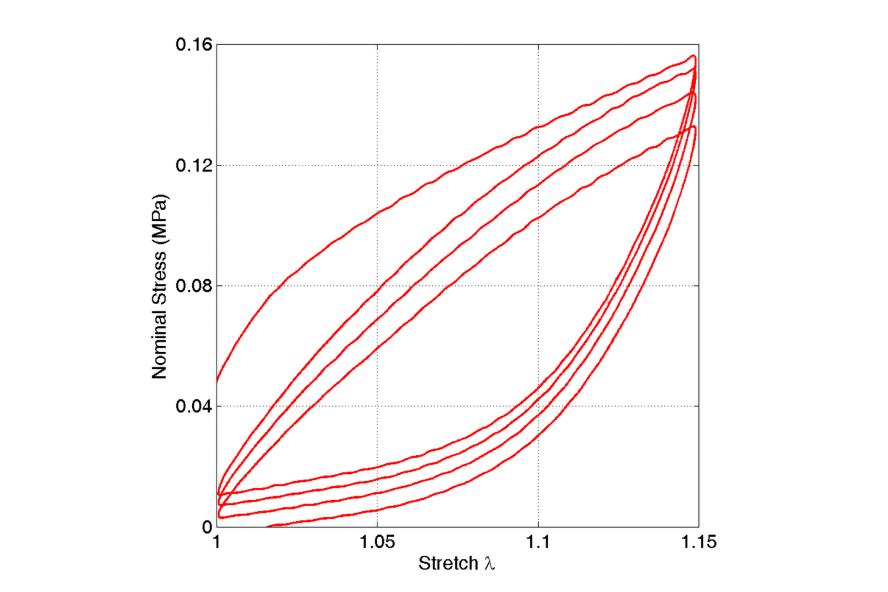
graph showing the pre-conditioning of a caterpillar muscle in the active state with maximum stretch of 1.15

Figure 3: Pre-conditioning of a caterpillar muscle in the active state with maximum stretch of λ = 1.15. Data for the stimulated condition were obtained during the final 8 seconds of a 10 seconds stimulus, corresponding to the period during which the same muscle preparation sustained force within 90 % of peak values under tetanic isometric stimulation.

In this project we examine the mechanical properties of muscles in a soft-bodied arthropod under both passive and stimulated conditions. In particular, we examine the ventral interior lateral muscle of the tobacco hornworm caterpillar, Manduca sexta, and show that its response is qualitatively similar to the behavior of particle reinforced rubber. Both materials are capable of large nonlinear elastic deformations, show a hysteretic behavior and display stress softening during the first few cycles of repeated loading. The Manduca muscle can therefore be considered as different elastic materials during loading and unloading, and is best described using the theory of pseudo-elasticity. There is, however, one important difference. Biological tissues are composed of an isotropic matrix embedding multiple oriented families of protein fibers. Therefore, the material response is best described as anisotropic. Rubber response, on the other hand, is generally isotropic.

We develop a constitutive model to describe the nonlinear mechanical response of a preconditioned Manduca muscle both for the passive and the stimulated conditions. The muscle consists of a microfibrillar matrix material with an embedded fibrous network of elastic proteins and the proposed model accounts for its nonlinear mechanical properties, including finite deformation pseudo-elasticity, anisotropy and energy dissipation associated with hysteresis. We further assume incompressibility since changes in volume for deformations within the physiological range are infinitesimal and therefore negligible. The model is fitted to available data and its predictions are assessed.

Publications

Ahamed T, Rubin MB, Trimmer BA, Dorfmann L (2016) The time-dependent behavior of passive skeletal muscle. Continuum Mech Therm 28:561-577.
Paetsch C, Dorfmann L (2015) Stability of active muscle tissue. J Eng Math 95:193-216.
Paetsch C, Dorfmann A (2013) Non-linear modeling of active biohybrid materials. Int J Nonlinear Mech 56:105-114.
Paetsch C, Trimmer BA, Dorfmann A (2012) A constitutive model for active-passive transition of muscle fibers. Int J Nonlinear Mech 47:377-387.
Lin HT, Paetsch CR, Slate D, Dorfmann L, Trimmer BA (2011) Ontogenetic scaling of caterpillar body properties and its biomechanical implications on the use of hydrostatic skeleton. J Exp Biol 214:1194-1204.
Lin HT, Dorfmann A, Trimmer BA (2009) Soft Cuticle Biomechanics: A Constitutive Model of Anisotropy for Caterpillar Integument. J Theor Biol 256:447-457.
Bose K., Dorfmann A (2009) Computational Aspects of a Pseudo-Elastic Constitutive Model for Muscle Properties in a Soft-Bodied Arthropod. International Journal of Non-Liner Mechanics 44:42-50.
Dorfmann A, Trimmer BA, Woods W.A (2008) Muscle Performance in a Soft-Bodied Terrestrial Crawler: Constitutive Modeling of Strain-Rate Dependency. J Roy Soc Interface 5:349-362.
Dorfmann A, Trimmer BA, Woods W.A (2007) A Constitutive Model for Muscle Properties in a Soft Bodied Arthropod. J Roy Soc Interface 4:257-269.